RS Aggarwal Class 9 Chapter Lines & Angles Exercise 4D Solution

EXERCISE 4D

Question 1:
Since, sum of the angles of a triangle is 180^o
∠A + ∠B + ∠C = 180^o
⇒ ∠A + 76^o + 48^o = 180^o
⇒ ∠A = 180^o – 124^o = 56^o
∴ ∠A = 56^o

Question 2:
Let the measures of the angles of a triangle are (2x)^o, (3x)^o and (4x)^o.
Then, 2x + 3x + 4x = 180 [sum of the angles of a triangle is 180^o ]
⇒ 9x = 180
⇒ x = \(\frac { 180 }{ 9 } \) = 20
∴ The measures of the required angles are:
2x = (2 × 20)^o = 40^o
3x = (3 × 20)^o = 60^o
4x = (4 × 20)^o = 80^o

Question 3:
Let 3∠A = 4∠B = 6∠C = x (say)
Then, 3∠A = x
⇒ ∠A = \(\frac { x }{ 3 } \)
4∠B = x
⇒ ∠B = \(\frac { x }{ 4 } \)
and 6∠C = x
⇒ ∠C = \(\frac { x }{ 6 } \)
As ∠A + ∠B + ∠C = 180^o

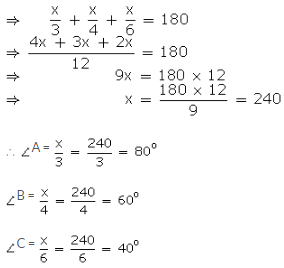
Question 4:
∠A + ∠B = 108^o [Given]
But as ∠A, ∠B and ∠C are the angles of a triangle,
∠A + ∠B + ∠C = 180^o
⇒ 108^o + ∠C = 180^o
⇒ C = 180^o – 108^o = 72^o
Also, ∠B + ∠C = 130^o [Given]
⇒ ∠B + 72^o = 130^o
⇒ ∠B = 130^o – 72^o = 58^o
Now as, ∠A + ∠B = 108^o
⇒ ∠A + 58^o = 108^o
⇒ ∠A = 108^o – 58^o = 50^o
∴ ∠A = 50^o, ∠B = 58^o and ∠C = 72^o.

Question 5:
Since. ∠A , ∠B and ∠C are the angles of a triangle .
So, ∠A + ∠B + ∠C = 180^o
Now, ∠A + ∠B = 125^o [Given]
∴ 125^o + ∠C = 180^o
⇒ ∠C = 180^o – 125^o = 55^o
Also, ∠A + ∠C = 113^o [Given]
⇒ ∠A + 55^o = 113^o
⇒ ∠A = 113^o – 55^o = 58^o
Now as ∠A + ∠B = 125^o
⇒ 58^o + ∠B = 125^o
⇒ ∠B = 125^o – 58^o = 67^o
∴ ∠A = 58^o, ∠B = 67^o and ∠C = 55^o.

Question 6:
Since, ∠P, ∠Q and ∠R are the angles of a triangle.
So, ∠P + ∠Q + ∠R = 180^o ….(i)
Now, ∠P – ∠Q = 42^o [Given]
⇒ ∠P = 42^o + ∠Q ….(ii)
and ∠Q – ∠R = 21^o [Given]
⇒ ∠R = ∠Q – 21^o ….(iii)
Substituting the value of ∠P and ∠R from (ii) and (iii) in (i), we get,
⇒ 42^o + ∠Q + ∠Q + ∠Q – 21^o = 180^o
⇒ 3∠Q + 21^o = 180^o
⇒ 3∠Q = 180^o – 21^o = 159^o
∠Q = \(\frac { 159 }{ 3 } \) = 53^o
∴ ∠P = 42^o + ∠Q
= 42^o + 53^o = 95^o
∠R = ∠Q – 21^o
= 53^o – 21^o = 32^o
∴ ∠P = 95^o, ∠Q = 53^o and ∠R = 32^o.

Question 7:
Given that the sum of the angles A and B of a ABC is 116^o, i.e., ∠A + ∠B = 116^o.
Since, ∠A + ∠B + ∠C = 180^o
So, 116^o + ∠C = 180^o
⇒ ∠C = 180^o – 116^o = 64^o
Also, it is given that:
∠A – ∠B = 24^o
⇒ ∠A = 24^o + ∠B
Putting, ∠A = 24^o + ∠B in ∠A + ∠B = 116^o, we get,
⇒ 24^o + ∠B + ∠B = 116^o
⇒ 2∠B + 24^o = 116^o
⇒ 2∠B = 116^o – 24^o = 92^o
∠B = \(\frac { 92 }{ 2 } \) = 46^o
Therefore, ∠A = 24^o + 46^o = 70^o
∴ ∠A = 70^o, ∠B = 46^o and ∠C = 64^o.

Question 8:
Let the two equal angles, A and B, of the triangle be x^o each.
We know,
∠A + ∠B + ∠C = 180^o
⇒ x^o + x^o + ∠C = 180^o
⇒ 2x^o + ∠C = 180^o ….(i)
Also, it is given that,
∠C = x^o + 18^o ….(ii)
Substituting ∠C from (ii) in (i), we get,
⇒ 2x^o + x^o + 18^o = 180^o
⇒ 3x^o = 180^o – 18^o = 162^o
x = \(\frac { 162 }{ 3 } \) = 54^o
Thus, the required angles of the triangle are 54^o, 54^o and x^o + 18^o = 54^o + 18^o = 72^o.

Question 9:
Let ∠C be the smallest angle of ABC.
Then, ∠A = 2∠C and B = 3∠C
Also, ∠A + ∠B + ∠C = 180^o
⇒ 2∠C + 3∠C + ∠C = 180^o
⇒ 6∠C = 180^o
⇒ ∠C = 30^o
So, ∠A = 2∠C = 2 (30^o) = 60^o
∠B = 3∠C = 3 (30^o) = 90^o
∴ The required angles of the triangle are 60^o, 90^o, 30^o.

Question 10:
Let ABC be a right angled triangle and ∠C = 90^o
Since, ∠A + ∠B + ∠C = 180^o
⇒ ∠A + ∠B = 180^o – ∠C = 180^o – 90^o = 90^o
Suppose ∠A = 53^o
Then, 53^o + ∠B = 90^o
⇒ ∠B = 90^o – 53^o = 37^o
∴ The required angles are 53^o, 37^o and 90^o.

Question 11:
Let ABC be a triangle.
Given, ∠A + ∠B = ∠C
We know, ∠A + ∠B + ∠C = 180^o
⇒ ∠C + ∠C = 180^o
⇒ 2∠C = 180^o
⇒ ∠C = \(\frac { 180 }{ 2 } \) = 90^o
So, we find that ABC is a right triangle, right angled at C.

Question 12:
Given : ∆ABC in which ∠A = 90^o, AL ⊥ BC
To Prove: ∠BAL = ∠ACB
Proof :
In right triangle ∆ABC,
⇒ ∠ABC + ∠BAC + ∠ACB = 180^o
⇒ ∠ABC + 90^o + ∠ACB = 180^o
⇒ ∠ABC + ∠ACB = 180^o – 90^o
∴ ∠ABC + ∠ACB = 90^o
⇒ ∠ ACB = 90^o – ∠ABC ….(1)
Similarly since ∆ABL is a right triangle, we find that,
∠BAL = 90^o – ∠ABC …(2)
Thus from (1) and (2), we have
∴ ∠BAL = ∠ACB (Proved)

Question 13:
Let ABC be a triangle.
So, ∠A < ∠B + ∠C
Adding A to both sides of the inequality,
⇒ 2∠A < ∠A + ∠B + ∠C
⇒ 2∠A < 180^o [Since ∠A + ∠B + ∠C = 180^o]
⇒ ∠A < \(\frac { 180 }{ 2 } \) = 90^o
Similarly, ∠B < ∠A + ∠C
⇒ ∠B < 90^o
and ∠C < ∠A + ∠B
⇒ ∠C < 90^o
∆ABC is an acute angled triangle.

Question 14:
Let ABC be a triangle and ∠B > ∠A + ∠C
Since, ∠A + ∠B + ∠C = 180^o
⇒ ∠A + ∠C = 180^o – ∠B
Therefore, we get
∠B > 180^o – ∠B
Adding ∠B on both sides of the inequality, we get,
⇒ ∠B + ∠B > 180^o – ∠B + ∠B
⇒ 2∠B > 180^o
⇒ ∠B > \(\frac { 180 }{ 2 } \) = 90^o
i.e., ∠B > 90^o which means ∠B is an obtuse angle.
∆ABC is an obtuse angled triangle.

Question 15:
Since ∠ACB and ∠ACD form a linear pair.
So, ∠ACB + ∠ACD = 180^o
⇒ ∠ACB + 128^o = 180^o
⇒ ∠ACB = 180^o – 128 = 52^o
Also, ∠ABC + ∠ACB + ∠BAC = 180^o
⇒ 43^o + 52^o + ∠BAC = 180^o
⇒ 95^o + ∠BAC = 180^o
⇒ ∠BAC = 180^o – 95^o = 85^o
∴ ∠ACB = 52^o and ∠BAC = 85^o.

Question 16:
As ∠DBA and ∠ABC form a linear pair.
So, ∠DBA + ∠ABC = 180^o
⇒ 106^o + ∠ABC = 180^o
⇒ ∠ABC = 180^o – 106^o = 74^o
Also, ∠ACB and ∠ACE form a linear pair.
So, ∠ACB + ∠ACE = 180^o
⇒ ∠ACB + 118^o = 180^o
⇒ ∠ACB = 180^o – 118^o = 62^o
In ∠ABC, we have,
∠ABC + ∠ACB + ∠BAC = 180^o
74^o + 62^o + ∠BAC = 180^o
⇒ 136^o + ∠BAC = 180^o
⇒ ∠BAC = 180^o – 136^o = 44^o
∴ In triangle ABC, ∠A = 44^o, ∠B = 74^o and ∠C = 62^o

Question 17:
(i) ∠EAB + ∠BAC = 180^o [Linear pair angles]

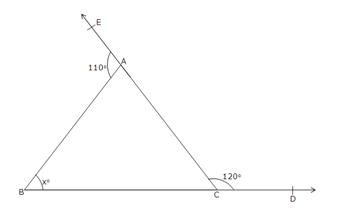
110^o + ∠BAC = 180^o
⇒ ∠BAC = 180^o – 110^o = 70^o
Again, ∠BCA + ∠ACD = 180^o [Linear pair angles]
⇒ ∠BCA + 120^o = 180^o
⇒ ∠BCA = 180^o – 120^o = 60^o
Now, in ∆ABC,
∠ABC + ∠BAC + ∠ACB = 180^o
x^o + 70^o + 60^o = 180^o
⇒ x + 130^o = 180^o
⇒ x = 180^o – 130^o = 50^o
∴ x = 50
(ii)

In ∆ABC,
∠A + ∠B + ∠C = 180^o
⇒ 30^o + 40^o + ∠C = 180^o
⇒ 70^o + ∠C = 180^o
⇒ ∠C = 180^o – 70^o = 110^o
Now ∠BCA + ∠ACD = 180^o [Linear pair]
⇒ 110^o + ∠ACD = 180^o
⇒ ∠ACD = 180^o – 110^o = 70^o
In ∆ECD,
⇒ ∠ECD + ∠CDE + ∠CED = 180^o
⇒ 70^o + 50^o + ∠CED = 180^o
⇒ 120^o + ∠CED = 180^o
∠CED = 180^o – 120^o = 60^o
Since ∠AED and ∠CED from a linear pair
So, ∠AED + ∠CED = 180^o
⇒ x^o + 60^o = 180^o
⇒ x^o = 180^o – 60^o = 120^o
∴ x = 120
(iii)

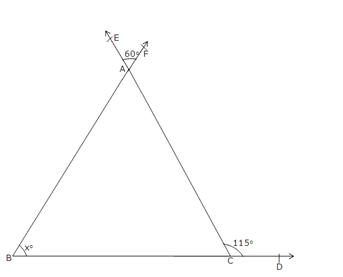
∠EAF = ∠BAC [Vertically opposite angles]
⇒ ∠BAC = 60^o
In ∆ABC, exterior ∠ACD is equal to the sum of two opposite interior angles.
So, ∠ACD = ∠BAC + ∠ABC
⇒ 115^o = 60^o + x^o
⇒ x^o = 115^o – 60^o = 55^o
∴ x = 55
(iv)

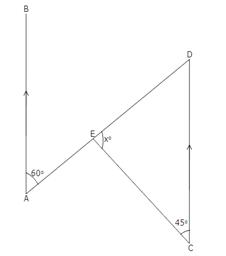
Since AB || CD and AD is a transversal.
So, ∠BAD = ∠ADC
⇒ ∠ADC = 60^o
In ∠ECD, we have,
∠E + ∠C + ∠D = 180^o
⇒ x^o + 45^o + 60^o = 180^o
⇒ x^o + 105^o = 180^o
⇒ x^o = 180^o – 105^o = 75^o
∴ x = 75
(v)

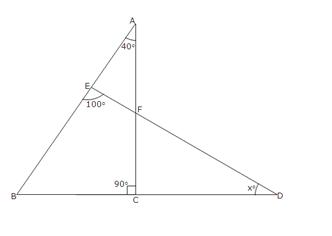
In ∆AEF,
Exterior ∠BED = ∠EAF + ∠EFA
⇒ 100^o = 40^o + ∠EFA
⇒ ∠EFA = 100^o – 40^o = 60^o
Also, ∠CFD = ∠EFA [Vertically Opposite angles]
⇒ ∠CFD = 60^o
Now in ∆FCD,
Exterior ∠BCF = ∠CFD + ∠CDF
⇒ 90^o = 60^o + x^o
⇒ x^o = 90^o – 60^o = 30^o
∴ x = 30
(vi)

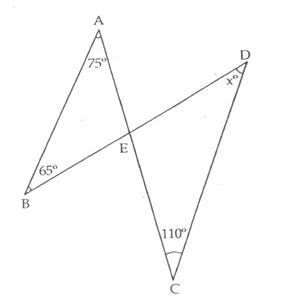
In ∆ABE, we have,
∠A + ∠B + ∠E = 180^o
⇒ 75^o + 65^o + ∠E = 180^o
⇒ 140^o + ∠E = 180^o
⇒ ∠E = 180^o – 140^o = 40^o
Now, ∠CED = ∠AEB [Vertically opposite angles]
⇒ ∠CED = 40^o
Now, in ∆CED, we have,
∠C + ∠E + ∠D = 180^o
⇒ 110^o + 40^o + x^o = 180^o
⇒ 150^o + x^o = 180^o
⇒ x^o = 180^o – 150^o = 30^o
∴ x = 30

Question 18:
Produce CD to cut AB at E.

Now, in ∆BDE, we have,
Exterior ∠CDB = ∠CEB + ∠DBE
⇒ x^o = ∠CEB + 45^o …..(i)
In ∆AEC, we have,
Exterior ∠CEB = ∠CAB + ∠ACE
= 55^o + 30^o = 85^o
Putting ∠CEB = 85^o in (i), we get,
x^o = 85^o + 45^o = 130^o
∴ x = 130

Question 19:
The angle ∠BAC is divided by AD in the ratio 1 : 3.
Let ∠BAD and ∠DAC be y and 3y, respectively.
As BAE is a straight line,
∠BAC + ∠CAE = 180^o [linear pair]
⇒ ∠BAD + ∠DAC + _∠CAE = 180^o
⇒ y + 3y + 108^o = 180^o
⇒ 4y = 180^o – 108^o = 72^o
⇒ y = \(\frac { 72 }{ 4 } \) = 18^o
Now, in ∆ABC,
∠ABC + ∠BCA + ∠BAC = 180^o
y + x + 4y = 180^o
[Since, ∠ABC = ∠BAD (given AD = DB) and ∠BAC = y + 3y = 4y]
⇒ 5y + x = 180
⇒ 5 × 18 + x = 180
⇒ 90 + x = 180
∴ x = 180 – 90 = 90

Question 20:
Given : A ∆ABC in which BC, CA and AB are produced to D, E and F respectively.
To prove : Exterior ∠DCA + Exterior ∠BAE + Exterior ∠FBD = 360^o
Proof : Exterior ∠DCA = ∠A + ∠B ….(i)
Exterior ∠FAE = ∠B + ∠C ….(ii)
Exterior ∠FBD = ∠A + ∠C ….(iii)
Adding (i), (ii) and (iii), we get,
Ext. ∠DCA + Ext. ∠FAE + Ext. ∠FBD
= ∠A + ∠B + ∠B + ∠C + ∠A + ∠C
= 2∠A + 2∠B + 2∠C
= 2 (∠A + ∠B + ∠C)
= 2 × 180^o
[Since, in triangle the sum of all three angle is 180^o]
= 360^o
Hence, proved.

Question 21:
In ∆ACE, we have,
∠A + ∠C + ∠E = 180^o ….(i)
In ∆BDF, we have,
∠B + ∠D + ∠F = 180^o ….(ii)
Adding both sides of (i) and (ii), we get,
∠A + ∠C +∠E + ∠B + ∠D + ∠F = 180^o + 180^o
⇒ ∠A + ∠B + ∠C + ∠D + ∠E + ∠F = 360^o.

Question 22:
Given : In ∆ABC, bisectors of ∠B and ∠C meet at O and ∠A = 70^o
In ∆BOC, we have,

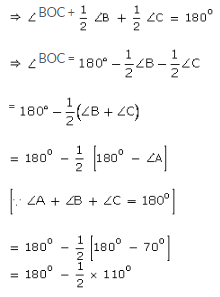
∠BOC + ∠OBC + ∠OCB = 180^o
= 180^o – 55^o = 125^o
∴ ∠BOC = 125^o.

Question 23:
We have a ∆ABC whose sides AB and AC have been procued to D and E. A = 40^o and bisectors of ∠CBD and ∠BCE meet at O.
In ∆ABC, we have,
Exterior ∠CBD = C + 40^o

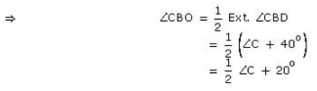
And exterior ∠BCE = B + 40^o

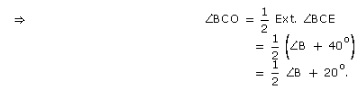
Now, in ∆BCO, we have,

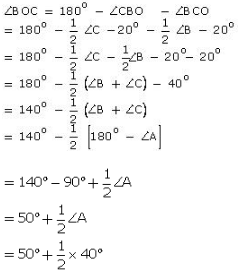
= 50^o + 20^o
= 70^o
Thus, ∠BOC = 70^o

Question 24:
In the given ∆ABC, we have,
∠A : ∠B : ∠C = 3 : 2 : 1
Let ∠A = 3x, ∠B = 2x, ∠C = x. Then,
∠A + ∠B + ∠C = 180^o
⇒ 3x + 2x + x = 180^o
⇒ 6x = 180^o
⇒ x = 30^o
∠A = 3x = 3 30^o = 90^o
∠B = 2x = 2 30^o = 60^o
and, ∠C = x = 30^o
Now, in ∆ABC, we have,
Ext ∠ACE = ∠A + ∠B = 90^o + 60^o = 150^o
∠ACD + ∠ECD = 150^o
⇒ ∠ECD = 150^o – ∠ACD
⇒ ∠ECD = 150^o – 90^o [since , AD ⊥ CD, ∠ACD = 90^o]
⇒ ∠ECD= 60^o

Question 25:
In ∆ABC, AN is the bisector of ∠A and AM ⊥ BC.
Now in ∆ABC we have;
∠A = 180^o – ∠B – ∠C
⇒ ∠A = 180^o – 65^o – 30^o
= 180^o – 95^o
= 85^o
Now, in ∆ANC we have;

Thus, ∠MAN =

Question 26:
(i) False (ii) True (iii) False (iv) False (v) True (vi) True.