RS Aggarwal Class 9 Math Chapter 2 Polynomials Exercise 2D Solution

EXERCISE 2D

Question 1:
f(x) = (x³ – 8)
By the Factor Theorem, (x – 2) will be a factor of f(x) if f(2) = 0.
Here, f(2) = (2)³ – 8
= 8 – 8 = 0
∴ (x – 2) is a factor of (x³ – 8).

Question 2:
f(x) = (2x³ + 7x² – 24x – 45)
By the Factor Theorem, (x – 3) will be a factor of f(x) if f(3) = 0.
Here, f(3) = 2 × 3³ + 7 × 3² – 24 × 3 – 45
= 54 + 63 – 72 – 45
= 117 – 117 = 0
∴ (x – 3) is a factor of (2x³ + 7x² – 24x – 45).

Question 3:
f(x) = (2x⁴ + 9x³ + 6x² – 11x – 6)
By the Factor Theorem, (x – 1) will be a factor of f(x) if f(1) = 0.
Here, f(1) = 2 × 1⁴ + 9 × 1³ + 6 × 1² – 11 × 1 – 6
= 2 + 9 + 6 – 11 – 6
= 17 – 17 = 0
∴ (x – 1) is factor of (2x⁴ + 9x³ + 6x² – 11x – 6).

Question 4:
f(x) = (x⁴ – x² – 12)
By the Factor Theorem, (x + 2) will be a factor of f(x) if f(-2) = 0.
Here, f(-2) = (-2)⁴ – (-2)² – 12
= 16 – 4 – 12
= 16 – 16 = 0
∴ (x + 2) is a factor of (x⁴ – x² – 12).

Question 5:
f(x) = 2x³ + 9x² – 11x – 30
By the Factor Theorem, (x + 5) will be a factor of f(x) if f(-5) = 0.
Here, f(-5) = 2(-5)³ + 9(-5)² – 11(-5) – 30
= -250 + 225 + 55 – 30
= -280 + 280 = 0
∴ (x + 5) is a factor of (2x³ + 9x² – 11x – 30).

Question 6:
f(x) = (2x⁴ + x³ – 8x² – x + 6)
By the Factor Theorem, (x – a) will be a factor of f(x) if f(a) = 0.
Here, 2x – 3 = 0 ⇒ x = \(\frac { 3 }{ 2 } \)

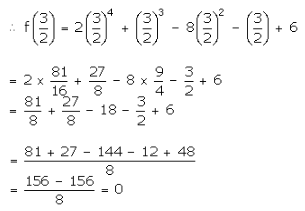
∴ (2x – 3) is a factor of (2x⁴ + x³ – 8x² – x + 6).

Question 7:
f(x) = (7x² – \(4\sqrt { 2 } \) x – 6 = 0)
By the Factor Theorem, (x – a) will be a factor of f(x) if f(a) = 0.
Here,
= 14 – 8 – 6
= 14 – 14 = 0
∴ (x – \(\sqrt { 2 } \)) is a factor of (7 – \(4\sqrt { 2 } \) x – 6 = 0).

Question 8:

f(x) = (\(4\sqrt { 2 } \)x² + 5x +\(\sqrt { 2 } \) = 0)
By the Factor Theorem, (x – a) will be a factor of f(x) if f(a) = 0.
Here,
∴ (x + \(\sqrt { 2 } \)) is a factor of (\(4\sqrt { 2 } \)x² + 5x +\(\sqrt { 2 } \) = 0).

Question 9:
f(x) = (2x³ + 9x² + x + k)
x – 1 = 0 ⇒ x = 1
∴ f(1) = 2 × 1³ + 9 × 1² + 1 + k
= 2 + 9 + 1 + k
= 12 + k
Given that (x – 1) is a factor of f(x).
By the Factor Theorem, (x – a) will be a factor of f(x) if f(a) = 0 and therefore f(1) = 0.
⇒ f(1) = 12 + k = 0
⇒ k = -12.

Question 10:
f(x) = (2x³ – 3x² – 18x + a)
x – 4 = 0 ⇒ x = 4
∴ f(4) = 2(4)³ – 3(4)² – 18 × 4 + a
= 128 – 48 – 72 + a
= 128 – 120 + a
= 8 + a
Given that (x – 4) is a factor of f(x).
By the Factor Theorem, (x – a) will be a factor of f(x) if f(a) = 0 and therefore f(4) = 0.
⇒ f(4) = 8 + a = 0
⇒ a = -8

Question 11:
f(x) = x⁴ – x³ – 11x² – x + a
x + 3 = 0 ⇒ x = -3
∴ f(-3) = (-3)⁴ – (-3)³ -11 (-3)² – (-3) + a
= 81 + 27 – 11 × 9 + 3 + a
= 81 + 27 – 99 + 3 + a
= 111 – 99 + a
= 12 + a
Given that f(x) is divisible by (x + 3), that is (x+3) is a factor of f(x).
By the Factor Theorem, (x – a) will be a factor of f(x) if f(a) = 0 and therefore f(-3) = 0.
⇒ f(-3) = 12 + a =0
⇒ a = -12.

Question 12:
f(x) = (2x³ + ax² + 11x + a + 3)
2x – 1 = 0 ⇒ x = \(\frac { 1 }{ 2 } \)
Given that f(x) is exactly divisible by (2x – 1), that is (2x – 1) is a factor of f(x).
By the Factor Theorem, (x – a) will be a factor of f(x) if f(a) = 0
and therefore \(f\left( \frac { 1 }{ 2 } \right) \) ≠ 0.
Therefore, we have

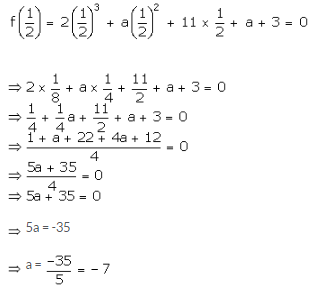
∴ The value of a = -7.

Question 13:
Let f(x) = (x³ – 10x² + ax + b), then by factor theorem
(x – 1) and (x – 2) will be factors of f(x) if f(1) = 0 and f(2) = 0.
f(1) = 1³ – 10 1² + a 1 + b = 0
⇒ 1 – 10 + a + b = 0
⇒ a + b = 9 ….(i)
And f(2) = 2³ – 10 2² + a 2 + b = 0
⇒ 8 – 40 + 2a + b = 0
⇒ 2a + b = 32 ….(ii)
Subtracting (i) from (ii), we get
a = 23
Substituting the value of a = 23 in (i), we get
⇒ 23 + b = 9
⇒ b = 9 – 23
⇒ b = -14
∴ a = 23 and b = -14.

Question 14:
Let f(x) = (x⁴ + ax³ – 7x² – 8x + b)
Now, x + 2 = 0 x = -2 and x + 3 = 0 x = -3
By factor theorem, (x + 2) and (x + 3) will be factors of f(x) if f(-2) = 0 and f(-3) = 0
∴ f(-2) = (-2)⁴ + a (-2)³ – 7 (-2)² – 8 (-2) + b = 0
⇒ 16 – 8a – 28 + 16 + b = 0
⇒ -8a + b = -4
⇒ 8a – b = 4 ….(i)
And, f(-3) = (-3)⁴ + a (-3)³ – 7 (-3)² – 8 (-3) + b = 0
⇒ 81 – 27a – 63 + 24 + b = 0
⇒ -27a + b = -42
⇒ 27a – b = 42 ….(ii)
Subtracting (i) from (ii), we get,
19a = 38
So, a = 2
Substituting the value of a = 2 in (i), we get
8(2) – b = 4
⇒ 16 – b = 4
⇒ -b = -16 + 4
⇒ -b = -12
⇒ b = 12
∴ a = 2 and b = 12.

Question 15:
Let f(x) = x³ – 3x² – 13x + 15
Now, x² + 2x – 3 = x² + 3x – x – 3
= x (x + 3) – 1 (x + 3)
= (x + 3) (x – 1)
Thus, f(x) will be exactly divisible by x² + 2x – 3 = (x + 3) (x – 1) if (x + 3) and (x – 1) are both factors of f(x), so by factor theorem, we should have f(-3) = 0 and f(1) = 0.
Now, f(-3) = (-3)³ – 3 (-3)² – 13 (-3) + 15
= -27 – 3 × 9 + 39 + 15
= -27 – 27 + 39 + 15
= -54 + 54 = 0
And, f(1) = 1³ – 3 × 1² – 13 × 1 + 15
= 1 – 3 – 13 + 15
= 16 – 16 = 0
∴ f(-3) = 0 and f(1) = 0
So, x² + 2x – 3 divides f(x) exactly.

Question 16:
Let f(x) = (x³ + ax² + bx + 6)
Now, by remainder theorem, f(x) when divided by (x – 3) will leave a remainder as f(3).
So, f(3) = 3³ + a × 3² + b × 3 + 6 = 3
⇒ 27 + 9a + 3b + 6 = 3
⇒ 9a + 3b + 33 = 3
⇒ 9a + 3b = 3 – 33
⇒ 9a + 3b = -30
⇒ 3a + b = -10 ….(i)
Given that (x – 2) is a factor of f(x).
By the Factor Theorem, (x – a) will be a factor of f(x) if f(a) = 0 and therefore f(2) = 0.
f(2) = 2³ + a × 2² + b × 2 + 6 = 0
⇒ 8 + 4a+ 2b + 6 = 0
⇒ 4a + 2b = -14
⇒ 2a + b = -7 ….(ii)
Subtracting (ii) from (i), we get,
⇒ a = -3
Substituting the value of a = -3 in (i), we get,
⇒ 3(-3) + b = -10
⇒ -9 + b = -10
⇒ b = -10 + 9
⇒ b = -1
∴ a = -3 and b = -1.